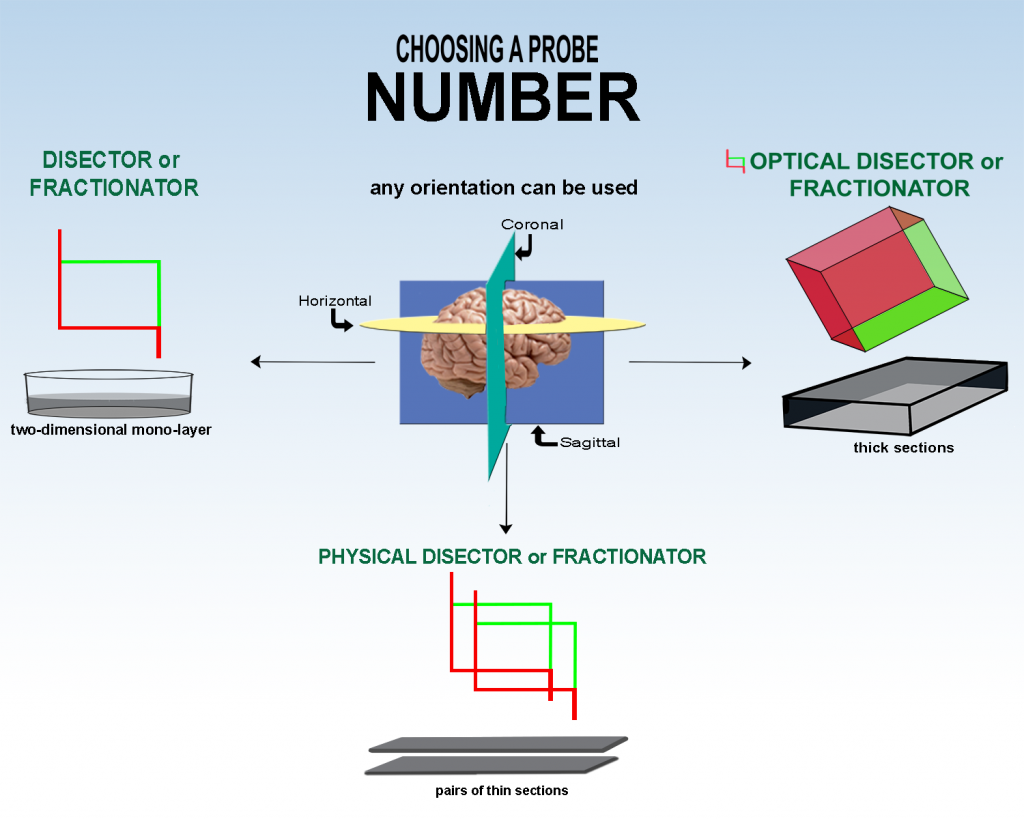
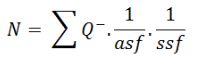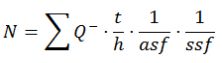The counting frame and systematic random sampling can be used to estimate the number of objects without bias. A very common example is the number of cells. Tissue sections of any orientation, including a preferred orientation may be used. This is because a unique point on the particle is counted, and a point looks the same from any direction. If you keep track of the volume fraction and extrapolate to get the estimate, it is called the fractionator method. If you calculate a numerical density it is called the disector method, an example of the NvVref method.
Disector or Fractionator
The number of objects in a flat region, for instance at the bottom of a petri dish can be estimated by using single counting frames (in the chart above, Disector refers to the NvVref method and Fractionator uses the fractionator method). Follow the counting frame rules. For the Disector, a numerical density is calculated and multiplied by the reference area. For the Fractionator, an extrapolation is done to arrive at an estimate (if you are working in one petri dish the ssf is one):
Physical Disector or Fractionator
To estimate number of objects in a three-dimensional region you need a three dimensional probe so that the leading edge of the particles can be identified. If you have thin sections (a couple of microns thick) you need to use pairs of thin sections (see Physical Disector for NvVref or Fractionator for the fractionator method in the chart above). If using the Physical Disector, a numerical density is multiplied by the reference volume. For the Physical Fractionator, the count is extrapolated:
Optical Disector or Fractionator
If you have thick sections you can use the Optical fractionator or Optical disector (see diagram above). The Optical Disector uses a numerical density that must be multiplied by a reference volume. Here is the equation for the Optical Fractionator:
D.C. Sterio, 1983, The Unbiased Estimation of Number and Sizes of Arbitrary Particles Using the Disector. Journal of Microscopy, 134, pp. 127-136
H.J.G. Gundersen, 1986, Stereology of Arbitrary Particles. Journal of Microscopy, 143, pp. 3-45
M.J. West, L. Slomianka, and H.J.G. Gundersen, 1991, Unbiased Stereological Estimation of the Total Number of Neurons in the Subdivisions of the Rat Hippocampus Using the Optical Fractionator. The Anatomical Record, 231, pp. 482-497
C.V. Howard and M.G. Reed, 2005, The 2D Fractionator, section 12.2, ‘Unbiased Stereology Second Edition’, Bios Scientific Publishers, NY, New York.
____________________________________________________________________

Sponsored by MBF Bioscience
developers of Stereo Investigator, the world’s most cited stereology system