What if the Tissue Sampled is Not Isotropic or Vertical?
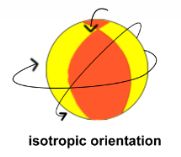
Ideally, probes that sample particles should be performed on isotropic or vertical sections, as indicated below, but this rule is often broken. If you use preferentially-oriented sections, the results will not be unbiased, but you can still get something out of this data. Plot the estimated areas in a histogram and report that these are biased estimates in that the orientation of the particles is not random. In other words, you tried to keep the sectioning in the same orientation for each animal (e.g. coronal), and you realize that over- or under-estimation of the real volumes or surfaces may occur due to the fact that every orientation in space is not a possibility for the particles. Of course if you use isotropic or vertical sectioning, you won’t have to worry about this bias.
Why not use Global Probes to Estimate Volume and Surface of Particles?
The problem with using the global probes Cavalieri/point-counting for particle volume estimates or Vertical Spatial Grid for particle surface estimates is that “there are serious practical problems at the top and the bottom of the cells. In these parts of the cell the measurements are difficult because the cell borders are cut tangentially by the observation plane and therefore have a fuzzy appearance.” (Tandrup, etal., 1997, pg. 108, last paragraph, third sentence). The local probes for particle-volume and particle-surface avoid these problems by not using information from the ambiguous tops and bottoms of particles. This is part of the reason that isotropic or vertical sections are required for the local particle probes, it ensures isotropy so that the same positions on the cells relative to their surroundings are not always the top and the bottom.
THREE FUNDAMENTALLY DIFFERENT WAYS TO SAMPLE PARTICLES
The particles for the study must be picked without bias or with known bias regarding size or orientation. This can be done, using systematic random sampling, in a number-weighted, a volume-weighted or a surface-weighted fashion. Volume weighted sampling means that larger cells are more likely to be sampled than those with a smaller volume (Sorensen, 1991, Fig. 9B). The mean volume weighted volume estimate is related to the mean number weighted volume (Gundersen and Jensen, 1985, equation 2):
V v = V 2 N / V N
V v is mean volume weighted volume
V N is mean number weighted volume
“It follows that V v > V N ” (Gundersen and Jensen, 1985, pg. 128, last sentence)
The method for selecting particles for a volume weighted estimate favors larger cells while that for picking particles for a number weighted estimate does not. A surface-weighted method of sampling is more likely to pick particles or holes that have a greater surface (Reed and Howard, 1988).
NUMBER WEIGHTED ESTIMATE
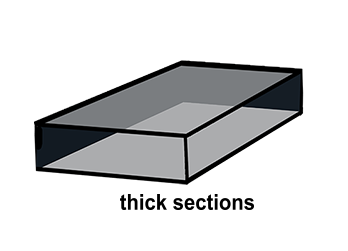
Use an optical disector and systematic random sampling to pick particles for a number weighted estimate. Follow the rules of the optical disector so that you pick the particles without bias regarding size or shape and then focus on a random spot in the middle of the particle. This is an example of using a local probe, the particle-estimator, during a global probe, the optical fractionator. Since an optical disector is being used the tissue sections must be thick:
The nucleator (Gundersen, 1988), planar rotator (Vedel Jensen and Gundersen, 1993), or optical rotator (Tandrup, etal., 1997) can be used to estimate the volume. The optical rotator or surfactor may be used to estimate surface.
VOLUME WEIGHTED ESTIMATE
Use Point-Sampled-Intercepts (Sorensen, 1991) sampling and systematic random sampling to select the cells. Unlike the nucleator/optical disector combination, Point-Sampled-Intercepts can be done on a thin section.
Parallel lines with equally spaced points are superimposed on the tissue. If a point falls on the cross section of a particle, that particle is selected; this is why cells with larger volumes are favored for selection. Point-Sampled-Intercepts sampling should be used when it is desired that the larger particles are studied (Gundersen etal., 1988, p. 863), for instance, when looking at the volume of nuclei in tumors cells (Sorenson, 1991; Arima, etal, 1997; Binder, etal., 1992).
SURFACE WEIGHTED ESTIMATE
The idea behind this type of sampling is to look at a particle or hole as it is ‘seen’ from the surface-element-boundary. Instead of point sampling to give a volume weighted estimate (above), line-segments are used. In the Gittes’ method (Reed and Howard, 1988, section 2.3), “an isotropic test system of cycloids is overlain on an isotropic uniform random plane”. As usual we recommend systematic random sampling. Either the cycloid grid or the image must be given an isotropic rotation. The cycloid line is used to probe for typical points on the surface. A surface point indicated by the cycloid is the starting point for a line segment, drawn to the next surface encountered. This line segment is drawn in the direction of the minor axis of the cycloid.
From these intercepts it is possible to calculate the surface weighted star volume, that can be used to think about a volume size from the ‘viewpoint’ of a surface that bounds that volume. For instance an alveoli as seen from the gas-exchange surface or a bone space as seen from it’s bone-wall.
Arima, K., Sugimura, Y., Hioki, T., Yamashita, A. and J. Kawamura (1997) Stereologically Estimated Mean Nuclear Volume of Prostatic Cancer is a Reliable Prognostic Parameter, British J. of Cancer, 76, 234 – 237.
Binder, M., Dolezal, I., Wolff, K., and H. Pehamberger (1992) Stereologic Estimation of Volume-Weighted Mean Nuclear Volume as a Predictor of Prognosis in “Thin” Malignant Melanoma
Gundersen H.J.G., and E.B. Jensen (1985) Stereological Estimation of the Volume-Weighted Mean Volume of Arbitrary Particles Observed on Random Sections, J. of Microscopy, 138, 127 – 142.
Gundersen, H.J.G. (1988) The Nucleator. J. of Microscopy, Vol. 151, pp. 3-21.
Reed, M.G. and C.V. Howard (1998) Surface-weighted Star Volume: Concept and Estimation, J. of Microscopy, 190, 350 – 356.
Sorenson F.B. (1991) Stereological Estimation of the Mean and Variance of Nuclear Volume from Vertical Sections, J. of Microscopy, 162, 203 – 229.
Tandrup, T., Gundersen, H.J.G. and E.B. Vedel Jensen (1997) The Optical Rotator. J. of Microscopy, 186, 108 -120.
Vedel Jensen, E.B. and H.J.G. Gundersen (1993) The Rotator. J. of Microscopy, 170, 35 – 44.
____________________________________________________________________

Sponsored by MBF Bioscience
developers of Stereo Investigator, the world’s most cited stereology system